The Marginal Tax Rate Barrier
In a future post, I will estimate how much extra funds the government could receive by raising taxes on upper income brackets. One might suppose that the maximum amount available is simply the total after-tax earnings of those brackets under current tax law. Here I will show that the limit is much lower because of the Marginal Tax Rate Barrier.
1. Constraints on Marginal Tax Rates
The tax rate is the percentage of before-tax income paid in tax. When a family earns additional income, the marginal tax rate is the additional tax paid, as a percentage of the additional income.
For instance, suppose there is a tax that is 0% for incomes up to $40k, and 10% for any additional income. One family making $50k and another making $80k both pay the same marginal tax rate (10%). The first family has a tax rate of 2% because the tax is 10% of $10k ($50k – $40k) which is $1k, or 2% of $50k. The second family’s tax rate is 5%. The current marginal tax rate for income tax is 37% for high income families, though less for capital gains income. Marginal rates as high as 91% in the 1950s and 70% in the 1970s.
It is obvious that a tax rate should be less than 100%, but there is a more stringent condition as well: the marginal tax rate should also be less than 100%. Otherwise, there is no point in earning any money subject to the tax, since it will all be taken by the government. As marginal tax rates approach 100%, people go to great lengths (both legal and illegal) to hide their money, or simply don’t bother earning the extra pre-tax income. In either case, such high marginal tax rates fail to collect more revenue for the government.
Similarly, the marginal tax rate should never be more than 100%. Suppose we want the tax to only affect families that have incomes greater than some lower threshold BL of their before-tax income. If a family earning more than BL has an additional tax rate x applied to their entire income, someone earning a dollar over BL would have to pay a much larger extra tax xBL, which would make their after-tax income decrease. The marginal tax rate would be greater than 100%, a great incentive to not report income above BL. The government can avoid this problem by applying the new tax only to income above BL. However, if the new tax falls only on income above a certain level, the marginal tax rate must be greater than the tax rate.
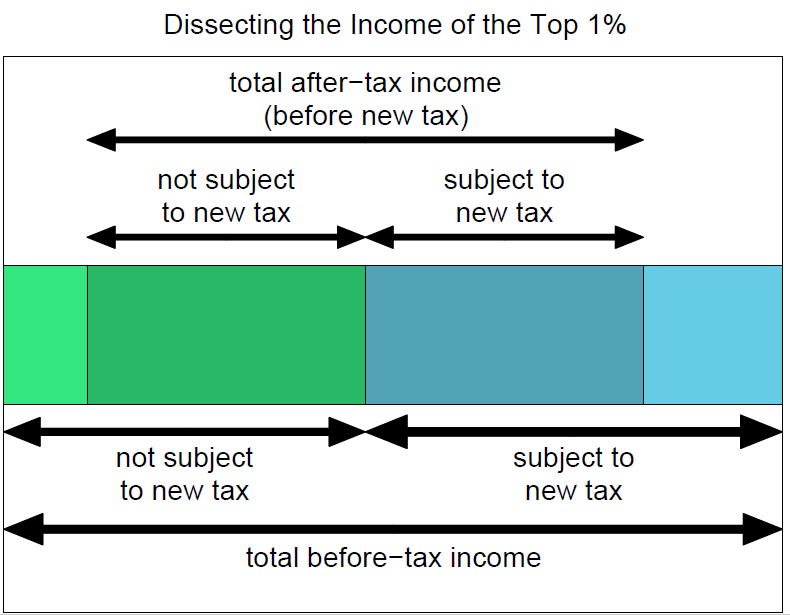
2. Summary of Result in a Single Graphic
Figure 1 illustrates the Marginal Tax Rate Barrier. The entire horizontal bar represents the income – before taxes – of some group of top earners, such as the top 1%. You can think of it as showing either an individual family income or the aggregated income of the entire group. A proposed new tax is only levied on income (shown in blue) above a certain level, BL, below which is income (shown in green) that will not be subject to additional tax. Each of these categories is divided between income left after taxes (dark shade), and income taken away as tax (light part).

1 Schematic of income segments.
How much can an additional tax take? Since by definition the new tax doesn’t apply to the green bars, the most it can take is 100% of the dark blue bar. Therefore the maximum possible increase in tax is not 100% of the after-tax income, but a much smaller amount.
3. Formula for Calculating the Rise in the Marginal Tax Rate
This section has some algebra which is inconvenient to write in the HTML post – if you’d like to see the math, please open the PDF version of this post.
4. Conclusion: Restricting New Taxes to Top Income Group Limits Taxes on the Group
I have shown that the amount of revenue that can be generated by increasing taxes on a group of top earners is limited by the Marginal Tax Rate Barrier. The need for marginal tax rates to be no more than 100% means that if the total after-tax income (based on old tax rates) is A and the after-taxes minimum income of the group is AL, then the most that can be generated is A-AL, or a fraction 1-r of A, where r=AL/A.
As the other post will show, the lower income limit for the top 1% of households is around one half the average income of households in the top 1%, so r is about .5 This is also approximately true for the top 5% and the top 20%. The current marginal tax rate for income tax is 37% for high income households, though less for capital gains income. Regardless of the current marginal rate, only half of the group’s income can be taxed if we want to shield everyone in lower brackets.
This may appear to generate a paradox: no matter what the initial marginal tax rate is, the same amount of additional taxes can be raised. However, if the initial tax rate were different, then the after-tax income A and perhaps r would be different as well. The formula derived here is diagnostic, not prescriptive.
Economic responses to high taxes will occur well before the tax rate hits the maximum, and of course there are political constraints as well. The calculation here suggests that these limiting effects on ability to collect tax will occur not as taxes approach 100% of the after-tax income, but when they approach a Marginal Tax Rate Barrier that may be about half as big.